“Even though most kids learn how to hold up to three or four by the time they go to kindergarten, there are some kids who don’t have the opportunities, and those kids have a really big problem in kindergarten,” said Art Parodi, a professor. children”. Emeritus at the University of Illinois at Urbana-Champaign, who has spent his career studying the best ways to teach counting, numbers, and arithmetic concepts to young children. “The reason for this is that dependency involves building an understanding of what is one, what is two, what is three, and what is four, which is the conceptual basis of number sense. There is a lot that depends on this understanding.
As children grow older, the process of subtitling can become more complex and useful for understanding more advanced math concepts. Imagine an array of seven points like this:
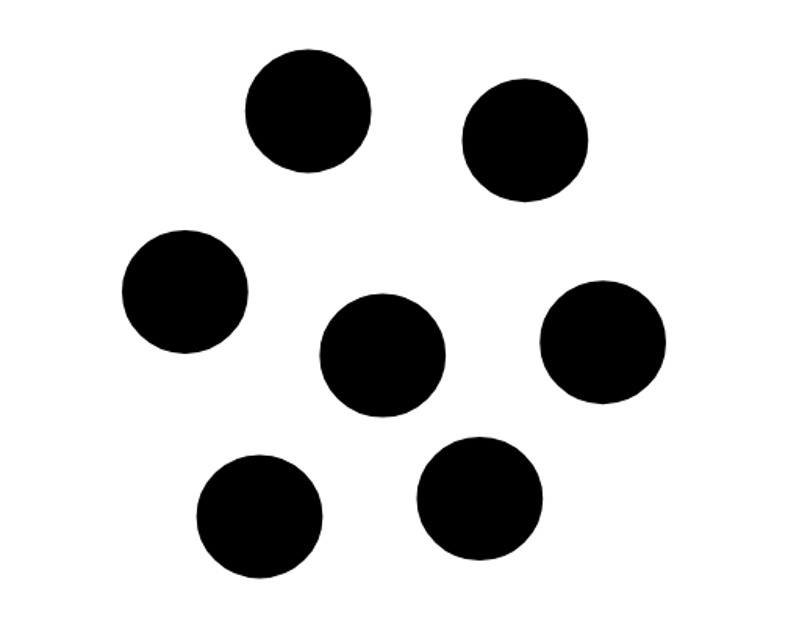
Most people cannot segment this many points, especially when they are not arranged in a familiar pattern, but they can detect subgroups. Some may see two sets of three plus an extra point. Others may see a group of four and a group of three. There are many possibilities.
Doug Clements and Julie Sarama, a research team at the University of Denver, call this quick division “conceptual division.” They say it helps children put together and break numbers, which is useful in learning addition and subtraction facts. For example, 7 + 5 can be a difficult sum to learn. But a child who can substitute may quickly see that the number 7 can be divided into 5 and 2. This makes it easier to add the two 5s together to get 10 plus 2 to get to 12, Clements said.
Counting by 7, of course, is another approach. But it may be mentally difficult to keep track of five more when a child is counting from 7 to 12. Children who know how to add only by counting soon realize that they do not have time to count when they are presented with a long addition worksheet. These children often memorize a series of meaningless numbers (5, 7, 12) which they soon forget. “They took the step of making it meaningful,” Clements said. “In first grade teachers will say, ‘He knew it last week.’ And now he’s forgotten it.’ Well, he didn’t really know that.”
Subitizing seems to help with all kinds of mathematical concepts, according to its supporters. In 2014, another group of researchers described how third graders who could quickly add groups of small numbers, such as three groups of two, had a more developed understanding of multiplication and could multiply faster. Some say that the ability to break a number into smaller, divisible parts builds an understanding of the relationship between the part and the whole and helps in working with fractions.
Clements and Sarama recommend using subitizing exercises until the end of elementary school. For example, a set of 24 dots can support conceptual understanding of multiplication in grades 3-5.
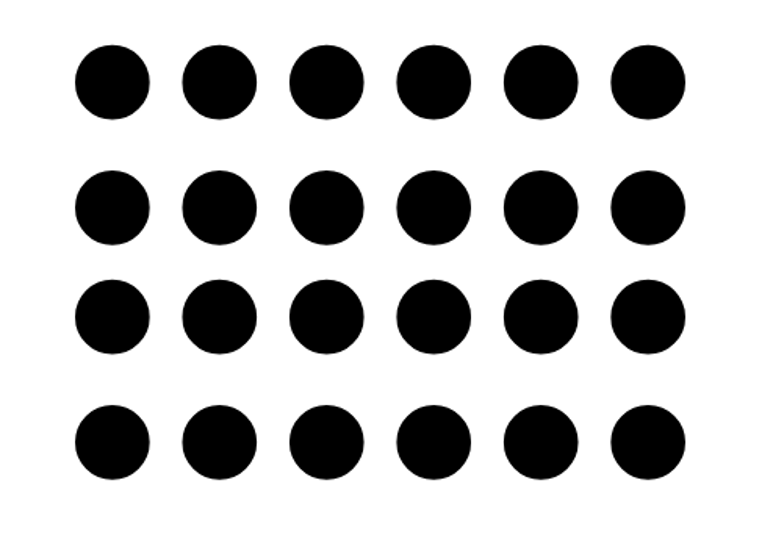
“Personalization is not a small thing you can move on from,” Clements said. “You just keep developing it in parallel with all the other skills you’re developing.”
Well, sometimes it’s actually kid stuff. Some researchers believe that babies as young as five or six months sort small numbers, such as two and three. This belief is based on experiments in which children held their gaze longer in response to changes in quantities. As children grow older, they begin to assign number words to groups of objects, for example, “two socks.” By age 2, many children can replace up to three items, and 4- and 5-year-olds can replace up to Three elements. Up to five items.
Researchers believe that enhancing this innate ability may help children build a sense of numbers. A case study of two students with mathematics difficulties, published in 2009, found that two-part conversion was helpful. Parodi of the University of Illinois promoted teaching subitizing in the 2013 Practice Guide on “Teaching Mathematics to Young Children,” for the Institute of Education Sciences, the research arm of the U.S. Department of Education.
Several studies have documented that children with stronger subscale abilities also tend to perform better in mathematics. For example, a 2022 study of more than 3,600 kindergartners in one southwestern school district found that children who were better at excelling also had significantly better math abilities in first grade. A 2020 study of 80 young children, ages 2 to 5, found that children who could skip the number four, and had learned to count, could usually answer the question “How many?” But children who could only surrender to the number three showed only a partial understanding of quantity.
However, a strong relationship between subconversion and mathematics ability is not evidence that students will benefit from subconversion instruction. It is possible that stronger donors will come from wealthier families who play more board games. These higher-income children also benefit from a range of other factors at home, from better nutrition to reduced stress, which can drive their success in math.
Empirical evidence demonstrating the importance of investing classroom time in the conversion process is scant, at least so far. In a new, but very small, study, 14 preschoolers ages 3 to 5 were randomly assigned to two different mathematics interventions. Those who were given more training on assignment performed better on the quantity comprehension test. The study, available online, is scheduled to be published in the December 2024 issue of the Journal of Sport Behavior. More larger studies are needed.
Even without this guide, many elementary school teachers have been incorporating aspects of personalization, sometimes called “quick pictures,” into their classrooms for decades. But more systematic teaching of the practice appears to be gaining popularity, based on interviews I conducted with teachers, researchers, and the National Council of Teachers of Mathematics, a professional organization. One motivation is the desire to address large achievement gaps between rich and poor children by building stronger mathematical foundations during the early years. Subconversion is explicitly included in the Illinois mathematics standards for kindergarten, while many other states suggest subconversion as an example of one way to teach numbers. Curriculum publishers are increasingly printing bitmap images for inclusion in their materials.
Transfer was a hot topic at the International Mathematics Education Conference earlier this summer, said Beth McDonald, an assistant professor of early childhood mathematics education at Illinois State University. “People come in and say, ‘Oh, we want to examine this,’” said MacDonald, who wrote her dissertation on subtitling more than a decade ago and is the author of several of the articles and substudies she read to write this article.
Although the assignment process is simple, experts say they sometimes see it taught incorrectly. One common mistake is asking students to count points. Researchers say this undermines the child’s confidence and ability to develop mental images of groups.
Speed is essential. Often the dots are displayed for a very long time, long enough for children to be able to count them. “That’s the biggest mistake,” said Clement’s partner, Sarama, who explained to me that brevity prompts children to visualize the picture themselves.
Each bullet exercise can be completed in 10 seconds for three minutes a day, Sarama says. Memory for quantities, according to the researchers, is built through short but repeated exposures.
According to Milwaukee math coach Robinson, kids want to talk so much about all the different ways they see points that it’s difficult for teachers to move on to the next activity. Children really enjoy translation.
Many children are learning how to get along at home. Researchers say this practice does not require fancy materials and parents do not need to create dot cards. They advise parents to talk about quantities during daily activities. When you’re folding laundry, talk about matching pairs of socks. Or at the end of lunch, ask who will eat the three fries left on the plate. Sometimes, you don’t have to count them!
